The role of pressure for incompressible flows
If we consider the continuity and momentum equations for a general compressible fluid:
![\frac{\partial \rho}{\partial t} +
\frac{\partial}{\partial x_j}\left[ \rho u_j \right] = 0](/Forums/vbLatex/img/1194ad2165bd70d6ccac3f24ea514126-1.gif "\frac{\partial \rho}{\partial t} +
\frac{\partial}{\partial x_j}\left[ \rho u_j \right] = 0")
![\frac{\partial}{\partial t}\left( \rho u_i \right) +
\frac{\partial}{\partial x_j}
\left[ \rho u_i u_j + p \delta_{ij} - \tau_{ji} \right] = 0](/Forums/vbLatex/img/5d8b30cb9598ed5bda495dcb5ce01e1d-1.gif "\frac{\partial}{\partial t}\left( \rho u_i \right) +
\frac{\partial}{\partial x_j}
\left[ \rho u_i u_j + p \delta_{ij} - \tau_{ji} \right] = 0")
taking the time derivative of the first one and the divergence of the second one:
![\frac{\partial^2 \rho}{\partial t^2} +
\frac{\partial}{\partial x_j}\left[\frac{\partial \left( \rho u_j \right)}{\partial t} \right] = 0](/Forums/vbLatex/img/6f2f3727f31ff6e507173603e8a990f6-1.gif "\frac{\partial^2 \rho}{\partial t^2} +
\frac{\partial}{\partial x_j}\left[\frac{\partial \left( \rho u_j \right)}{\partial t} \right] = 0")
![\frac{\partial}{\partial t}\left[\frac{\partial \left( \rho u_i \right)}{\partial x_i} \right] = -
\frac{\partial^2}{\partial x_i x_j}
\left[ \rho u_i u_j + p \delta_{ij} - \tau_{ji} \right]](/Forums/vbLatex/img/ab52f861fac8af8cf958682457e653ce-1.gif "\frac{\partial}{\partial t}\left[\frac{\partial \left( \rho u_i \right)}{\partial x_i} \right] = -
\frac{\partial^2}{\partial x_i x_j}
\left[ \rho u_i u_j + p \delta_{ij} - \tau_{ji} \right]")
and finally substituting the second in the first one, we get:
![\frac{\partial^2 \rho}{\partial t^2} =
\frac{\partial^2}{\partial x_i x_j}
\left[ \rho u_i u_j + p \delta_{ij} - \tau_{ji} \right]](/Forums/vbLatex/img/5fa795eb9d663dd3950c0b7a5ce1f803-1.gif "\frac{\partial^2 \rho}{\partial t^2} =
\frac{\partial^2}{\partial x_i x_j}
\left[ \rho u_i u_j + p \delta_{ij} - \tau_{ji} \right]")
with:
Now, considering single component flows, we have:
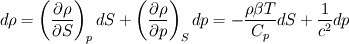
Obviously, an incompressible fluid doesn't even exists. For the present task we can assume the FLOW (not the fluid) as isothermal and we'll get (which, however, is the same of getting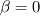
):
![\frac{1}{c^2} \frac{\partial^2 p}{\partial t^2} =
\frac{\partial^2}{\partial x_i x_j}
\left[ \rho u_i u_j + p \delta_{ij} - \tau_{ji} \right]](/Forums/vbLatex/img/af887cf59bc2f99ce4f4c9b32f442a50-1.gif "\frac{1}{c^2} \frac{\partial^2 p}{\partial t^2} =
\frac{\partial^2}{\partial x_i x_j}
\left[ \rho u_i u_j + p \delta_{ij} - \tau_{ji} \right]")
And finally moving all the pressure terms to the left side:
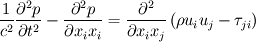
Considering the last equation (which, i think, should be correct) we can say something about the nature of the pressure.
In fact, mathematically speaking, it is similar (somehow and neglecting some lower order terms) to an externally forced mass-spring system with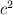
playing the role of k, the elastic costant of the spring.
So, what happens when k is very high?
In the mass spring-system, after some very fast oscillations (the more k is high the more they are faster), the mass will assume a displacement in equilibrium with the external forcing. The same is true even if the external forcing is not costant but relatively slow, and at every time the mass can be considered in equilibrium with the external force.
In this case the problem is called stiff because the time scale of the system is very different from that of the external forcing.
Going back to the pressure equation, the role of k is now played by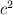, the speed of sound in the fluid. When 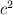is very high but the fluid velocity is relatively slow compared to 
, the problem becomes stiff, that is the thermodynamic is much more faster than the external forcing; this comes out nondimensionalising the right hand terms with 
.
Actually, in this case, the thermodynamic nature of the pressure is not changed (why should it be?) but (first of all for computational purposes) we can consider a new equation in which the time derivative term is omitted because of the
term (being of lower order):
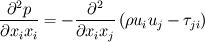
Moreover, with a very lenghty procedure, it can be shown that the divergence of the velocity field is proportional to terms involving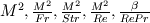
, so, in the same hypothesis already made, it also is of lower order and can be assumed to be zero, so we finally get:
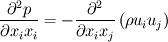
Which is of very different nature respect to the original one, being an equilibrium equation for the pressure (not a time-evolution one), so at every time the pressure is considered in equilibrium or, mathematically speaking, the pressure is considered acting as a Lagrange multiplier for the velocity field; in fact the equation is now a cinematic condition on the velocity field which has to be fulllfilled at every time.
So, what actually changes between the incompressible view and a general one, it's not the pressure nature, which is still thermodynamic, but the parameters affecting it. Actually, in the incompressible view, we are considering the pressure as affected by normal momentum fluxes only and, because the pressure fastly recovers the equilibrium after a momentum change (because of the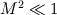
hypothesis), we just consider it as in equilibrium at each time omitting the lower order time-derivative term. As i said, this is much more a computational necessity (because of the stiffness); in fact, if you are interested in acoustic you will need to retain the time-derivative term and use one of the method of the computational aeroacoustic to treat the equation.
taking the time derivative of the first one and the divergence of the second one:
and finally substituting the second in the first one, we get:
with:
Now, considering single component flows, we have:
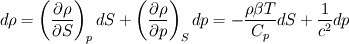
Obviously, an incompressible fluid doesn't even exists. For the present task we can assume the FLOW (not the fluid) as isothermal and we'll get (which, however, is the same of getting
):And finally moving all the pressure terms to the left side:
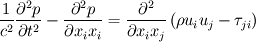
Considering the last equation (which, i think, should be correct) we can say something about the nature of the pressure.
In fact, mathematically speaking, it is similar (somehow and neglecting some lower order terms) to an externally forced mass-spring system with
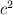playing the role of k, the elastic costant of the spring.So, what happens when k is very high?
In the mass spring-system, after some very fast oscillations (the more k is high the more they are faster), the mass will assume a displacement in equilibrium with the external forcing. The same is true even if the external forcing is not costant but relatively slow, and at every time the mass can be considered in equilibrium with the external force.
In this case the problem is called stiff because the time scale of the system is very different from that of the external forcing.
Going back to the pressure equation, the role of k is now played by
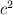, the speed of sound in the fluid. When 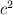is very high but the fluid velocity is relatively slow compared to , the problem becomes stiff, that is the thermodynamic is much more faster than the external forcing; this comes out nondimensionalising the right hand terms with .Actually, in this case, the thermodynamic nature of the pressure is not changed (why should it be?) but (first of all for computational purposes) we can consider a new equation in which the time derivative term is omitted because of the
term (being of lower order):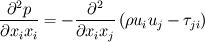Moreover, with a very lenghty procedure, it can be shown that the divergence of the velocity field is proportional to terms involving
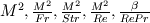, so, in the same hypothesis already made, it also is of lower order and can be assumed to be zero, so we finally get: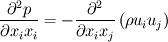Which is of very different nature respect to the original one, being an equilibrium equation for the pressure (not a time-evolution one), so at every time the pressure is considered in equilibrium or, mathematically speaking, the pressure is considered acting as a Lagrange multiplier for the velocity field; in fact the equation is now a cinematic condition on the velocity field which has to be fulllfilled at every time.
So, what actually changes between the incompressible view and a general one, it's not the pressure nature, which is still thermodynamic, but the parameters affecting it. Actually, in the incompressible view, we are considering the pressure as affected by normal momentum fluxes only and, because the pressure fastly recovers the equilibrium after a momentum change (because of the
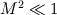hypothesis), we just consider it as in equilibrium at each time omitting the lower order time-derivative term. As i said, this is much more a computational necessity (because of the stiffness); in fact, if you are interested in acoustic you will need to retain the time-derivative term and use one of the method of the computational aeroacoustic to treat the equation.
Total Comments 0
