Centreline velocity in pipes
 

Dear all,
I have two questions...
1) How to calculate centreline velocity (Uc) in pipes? How's it different from mean velocity?
2) How to calculate turbulent boundary layer thickness?
Looking forward for you valuable answers.
Regards
Shahzad

Center-line velocity is usually the maximum velocity in the pipe, so it is, most of the time, larger than the average velocity.

Average velocity is easy to calculate:



so if you know the mass flow rate , the density , and the pipe cross-sectional area , you can calculate what the average velocity is.

Maximum velocity depends on the flow condition and geometry and .... For example, for a laminar circular-pipe flow, when the flow is fully-developed, maximum velocity is the same as center-line velocity, and is equal to twice the average velocity. Same conditions, but a rectangular duct with large aspect ratio will give you 1.5 times the average velocity.

For your second question, you need to provide a little bit more context. For example: what is the geometry?

Its a horizontal pipe. Re=35000. Basically its a highly turbulent flow. So in this case what would be the centreline velocity? Is there any pet formula to calculate it like average velocity you mentioned?
For question 2, I read in a book about boundary layer thickness its equal to delta(x)=0.37(x)(Ux/v) where v is kinematic viscosity.

As a rule of thumb you can use:



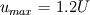for fully-developed, turbulent flows in a circular pipe. But the factor of 1.2 changes with Reynolds number. It should be okay for your case though. I remember there was a formula in Schlichting's "boundary-Layer Theory" book for the relationship between that factor and Reynolds number. You might be able to find it in other fluid mechanics text books as well. White's fluid mechanics book might have it too, but I'm not sure.

For the boundary layer thickness, the equation that you are talking about is for flat plate boundary layer (I think). If you want to apply it to pipe flow, it can only be used at the very beginning of the pipe where boundary layer thickness is much smaller than the pipe radius, so pipe wall is almost like a flat plate as far as the boundary layer is concerned. But as soon as the boundary layer thickness increases, it can "see" the curvature of the wall as well as the "free-stream" velocity change and therefor the pressure gradient, so the equation won't be valid anymore.

Yes you are right I have found the relation in a Fluid Mechanics book its something like this Umax=U(1+1.33sqrt(f)).
Regarding boundary layer thickness in turbulent pipe flow I am unable to find a relation. I was thinking if I replace x by D (pipe diameter) in the above relation it will work for pipe. I don't know whether this is correct or not. Could you guide me with this thickness relation or recommend me some books to get a relation.
Thanks

Boundary layer, in the sense that is defined in external flows, for a pipe grows when flow is developing, and as soon as it becomes fully-developed, then boundary layer thickness is equal to the pipe radius.

However, when you look at the velocity profile for a turbulent flow in a pipe, you can see few distinct regions. They are the viscous sub-layer, buffer layer, and log-law layer. I don't know if these are what you are looking for or the actual growth of the boundary layer thickness starting from a uniform velocity at inlet until the flow becomes fully developed?

If you are looking for the thickness of each of those three regions I mentioned, they are usually specified in a nondimensional coordinate system that originates on the wall (y+). The viscous sub-layer is usually y+ < 5, the log -law layer is usually y+ > 30~60, and the buffer layer is in between those.