CFD Online Discussion Forums - production term on staggered grid

Hi everybody,
I'm writing a code for simulation of a turbulent flow using finite volume, fully staggered grid and the standard k-epsilon equations. Staggered grids have the advantage of being conservative of kinetic energy , which becomes important for turbulent flows. But I have a problem in calculating the production term in the k and epsilon equations. As you know the production term involves calculating u and v gradients with respect to x and y at the center of the volumes. Now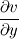, $\frac{\partial u}{\partial x}$ and $\frac{\partial v}{\partial y}$ can be easily found from central differencing:
$\frac{\partial u}{\partial x}=\frac{u_e-u_w}{\Delta x}$
$\frac{\partial v}{\partial y}=\frac{v_n-v_s}{\Delta y}$ ,:)
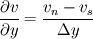but i can't find an easy way for finding 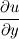$\frac{\partial u}{\partial y}$ and 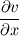$\frac{\partial v}{\partial x}$ , since we don't have the u velocities at north and south faces and v velocities at east and west faces.
Sure I can find these gradients on the corners of the volumes and then average them. But I don't think this is a systematic way and whether this will ruin the whole conservativeness property of the scheme??? :confused:
I have been thinking about this problem for several weeks now, but I think I lack the knowledge and experience for finding a goodway out of it. Any help is appreciated.