CFD Online Discussion Forums - Odd behaviour of the length

I am examining a 1D rod using mass co-ordinates under the effect of gravity. I have the rods on their end so that gravity will assist it. The equations are specific volume $\nu$ and u, the velocity. The mass co-ordinate is given by:
$h=\int_{0}^{x}\rho(t,s)ds$
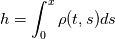
The equations are then:
$\frac{\partial\nu}{\partial t}=\frac{\partial u}{\partial h}$
$\frac{\partial u}{\partial t}=\frac{\partial}{\partial h}\left(\frac{\zeta(\nu)}{\nu}\frac{\partial u}{\partial h}\right)-g\nu$

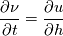
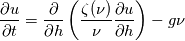
I'm unsure about what should happen. For the application, the length should monotonically decrease. Do you have any idea what's going on?
Code:
%this code solves the following system of equations:

%v_t=u_h

%u_t=(zeta/v*u_h)_h

%dX/dt=u

%h is defined by h_x=1/v, and X is the new position of the interfacial

%points

%The boundary conditions for u is u(t,0)=0, u_h(t,1)=-v(t,1)

%The BC's for v, can be derived from ones on u. 

%here u is the velocity, and v is the specific volume defined as 1/rho, where rho is the density 

%The system is in conservative form, and the finite volume method is the best method for these types of equations 

clear;clc;

S=struct;

%---define physical constants 

N=200; %This is the number of cells in the simulation

S.N=N;

S.alpha=0.2;

c_p=1; %heat capacity.

rho_half(1:N)=0.6; %This is the initial density

eta_0=0.005; 

S.eta=eta_0;

M=300; %This is the number of points in the time domain.

%---Set up geometry

x=linspace(0,1,N+1); %Initial spacing of the cell interfaces and ends.

dx=x(2); %initial lengths of cells

t=linspace(0,300,M); % This is the time of integration

L=zeros(M,1); %The length of the of the piece

L(1)=1; %Initial length

dt=t(2); %time increment

S.dt=dt;

mu=dt/dx^2;



%---Set up of variables

nu=zeros(M,N); %The specific volume =1/density

u=zeros(M,N); %The speed of the material

X=zeros(M,N+1); %The position of cell interfaces

X(1,:)=x; 



%---The solution of the system will be done via Newton-Raphson. The two

%variables v and u will be put into one vector y=[v u]' 

y_old=zeros(1,2*N); %This is the initial vector used.

nu(1,:)=1./rho_half; %Initial specific volume

y_old(1:N)=nu(1,:);

%v(1,:)=y_old(N+1:2*N);

theta=0.5-1/(12*mu); %This will meximise the timestep.

rho_0=zeros(1,N+1); %This sets up the calculation for h to begin with.

%This interpolates the density from the cell centres to cell boundaries.

rho_0(2:N)=0.5*(rho_half(1,N-1)+rho_half(2:N));

rho_0(1)=1.5*rho_half(1)-0.5*rho_half(2);

rho_0(N+1)=1.5*rho_half(N)-0.5*rho_half(N-1);

h=cumtrapz(x,rho_0); %calculates h, which is used throughout the timesteps.

nu_m(1:N)=1; %This is the matal powder density, the maximum possible density.

S.nu_m=nu_m;

dh=diff(h); %This is used to approximate the derivative

S.dh=dh;

tol=1e-5; %Tolerance on the Newton-Raphson method.

dx=diff(x);



for i=2:M

    y_init=1.001*y_old; %This is an initial guess for the new solution forbased upon the previous timestep solution

    y = Newton(tol,y_old,y_init,theta,S); %call the Newton Raphson routine

    nu(i,:)=y(1:N); %First N entries are the specific volume

    u(i,:)=y(N+1:2*N); %the second entries are the velocities

    %Compute the new grid

    dX=dh.*nu(i,:); %The mass in each cell in constant, use density*length=mass to compute the new length of the cell

    X(i,2:N+1)=cumsum(dX); %x(2:N+1)-(dx-dX); %Update the Eulerian co-ordinates. 

    L(i)=X(i,N+1); %The length of the rod is the last entry

    y_old=y; %Set the new solution as the old one.

end



plot(t,L)

r_half=1./nu(end,:);

X_half=0.5*(X(end,1:N)+X(end,2:N+1));

r=interp1(X_half,r_half,X(end,:));

v=interp1(X_half,u(end,:),X(end,:));

figure;

plot(X(end,:),r);

xlabel('X(t)')

ylabel('Density');

figure;

plot(X(end,:),v);

xlabel('X(t)')

ylabel('Velocity');

% function y=mass_flux(nu,u)

% global N

% global dh

% y=zeros(1,N+1);

% y(2:N)=0.5*(u(2:N)+u(1,N-1));

% y(N+1)=u(N)-0.25*dh(N)*(3*nu(N)-nu(N-1));

% end



function y=flux(u,nu,S)

%This computes the fluxes required for the finite volume method 

N=S.N;

dh=S.dh;

nu_0=S.nu_m;

k=0.01;

a=0.01;

nu_end=1.5*nu(N)-0.5*nu(N-1);

nu_0_end=1.5*nu_0(N)-0.5*nu_0(N-1);

y=zeros(2,N+1); %This vector will store the fluxes for the mass and momentum

y(1,2:N)=0.5*(u(2:N)+u(1,N-1)); %Fluxes for the mass

y(1,N+1)=u(N)-(nu_end*k/zeta(nu_end,nu_0_end,S))*dh(N)/2;

nu_face=0.5*(nu(1:N-1)+nu(2:N)); %This is specific volume on the cell interface

y(2,2:N)=P_L(a,nu_face,nu_0(2:N))+(4*zeta(nu_face,nu_0(2:N),S)./nu_face).*((u(2:N)-u(1:N-1))./(dh(2:N)+dh(1:N-1))); %The flux for the momentum equation

%nu_L=1.5*nu(1)-0.5*nu(1); %the specific volume at the end

nu_L=nu(1)+dh(1)*(nu(2)-nu(1))/(dh(1)+dh(2)); %The matal powder specific density  

%nu_0_L=1.5*nu_0(1)-0.5*nu_0(1); 

nu_0_L=nu_0(1)+dh(1)*(nu_0(2)-nu_0(1))/(dh(1)+dh(2));

y(2,1)=P_L(a,nu_L,nu_0_L)+(zeta(nu_L,nu_0_L,S)/nu_L)*(2*(u(2)-u(1))/(dh(1)+dh(2))); %the fluxes at the end

y(2,N+1)=0;

end



function y=P_L(a,nu,nu_0) %This is the laplace pressure 



    x=1-nu_0./nu; %This is the porosity for an imcompressible metal powder

    y=a*(1-x).^2;



end



function y=zeta(z,nu_0,S)

eta_0=S.eta;

  x=1-nu_0./z;

  y = 2*(1-x).^3*eta_0./(3*x);

end



function [J] = jacobian(y_old,y,theta,S)

% Computes the Jacobian matrix of the function f(x)

% f: function handle that returns a vector of function values

% x: column vector of independent variables



% Number of function values and independent variables

n = length(y); % number of independent variables



% Initialize Jacobian matrix

eps=1e-5; % could be made better

J = zeros(n,n);

T=zeros(1,n);

for i=1:n

    T(i)=1;

    fPlus = sintering_RHS(y_old,y+eps*T,theta,S);

    fMinus = sintering_RHS(y_old,y-eps*T,theta,S);

    J(:,i) = (fPlus-fMinus)/(2*eps);

    T(i)=0;

end

end



function f=sintering_RHS(y_0,y,theta,S)

%the vector f has the stencil for each cell for both the mass and momentum

nu_m=S.nu_m;

dt=S.dt;

N=floor(length(y)/2);

f=zeros(1,2*N);

flux_1=flux(y(N+1:2*N),y(1:N),S); %This is flux at timestep i 

flux_0=flux(y_0(N+1:2*N),y_0(1:N),S); %this is the flux at timestep i-1

f_mass=flux_1(1,:);

f_mom=flux_1(2,:);

f_mass_0=flux_0(1,:);

f_mom_0=flux_0(2,:);

f(1:N)=y(1:N)-y_0(1:N)-dt*theta*(f_mass(2:N+1)-f_mass(1:N))-(1-theta)*dt*(f_mass_0(2:N+1)-f_mass(1:N)); %These are the stencils coming from the system of equations 

f(N+1:2*N)=y(N+1:2*N)-y_0(N+1:2*N)-dt*theta*(f_mom(2:N+1)-f_mom(1:N)-S.alpha*y(1:N))-(1-theta)*dt*(f_mom_0(2:N+1)-f_mom_0(1:N)+S.alpha*y_0(1:N));

f(1)=y(1)-y(2);

end



function [y] = Newton(tol,y_old,y0,theta,S)

    iter = 0;

    maxiter = 10;

    error=10;

    J0 = jacobian(y_old,y0,theta,S); %Computes Jacobian

    J_inv=inv(J0);

    %[L,U] = lu(J0);

    while (error > tol) && (iter < maxiter)

        %J0 = jacobian(y_old,y0,theta); %Computes Jacobian

        f_old=sintering_RHS(y_old,y0,theta,S); %Computes the old f

        delta_y = -J_inv*f_old'; %Computes the differencebetween old and new guesses 

        y = y0 + delta_y'; %the new guess

        error=norm(sintering_RHS(y0,y,theta,S)); %computes the error, the error should be very small

        y0 = y;  

        

        iter = iter + 1; %Updates the iteration

%         fprintf('Iteration %i, norm = %.2f\n', iter, error)

    end

end