 |
|
|
[Sponsors] | |||||
|
|
|
#1 |
|
New Member
Join Date: Jul 2009
Location: near Marseille, France
Posts: 7
Rep Power: 17  |
Hello
I'd like to calculate the pressure variation between the inlet and the outlet of a heating channel. The causes of this variation are well known : - Friction, because of viscosity and turbulence : IRREVERSIBLE - Fluid Acceleration, either because of density change (heating for instance!) or because of cross section change : REVERSIBLE - Gravity, if z coordinate changes : REVERSIBLE Now I want to give an expression for these different components, but my two methods are not in agreement... 1) First Method: 1D Navier-Stokes 1D Momentum equation: 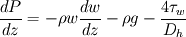 ( (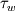 is wall friction, thus the whole term is the one responsible for friction pressure drop) is wall friction, thus the whole term is the one responsible for friction pressure drop)From which we define: 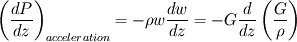 where 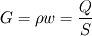 is mass velocity (Q is the mass flowrate and S is the cross section) is mass velocity (Q is the mass flowrate and S is the cross section)It comes: 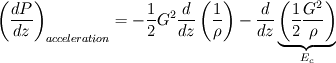 Then integration of a small portion of my channel gives: 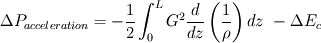 - When density is constant (no heating) and cross section varies: First term is zero, so that leaves us with 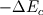 only. only.- When density changes but cross section is constant: G is also constant so that both terms are finally equal, and we obtain for the pressure drop 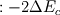 2) Second Method: Generalized Bernoulli's law Let's define hydraulic head, which represents the energy necessary to the fluid motion through piping: 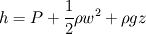 Generalized Bernoulli's law yields in: 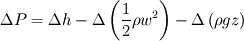 We recognize: a) 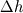 : Head loss due to friction : Head loss due to frictionb) 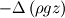 : Pressure drop due to gravity : Pressure drop due to gravityBut then, what is 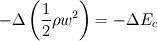 ?? It is indeed pressure drop due to acceleration of the fluid, in the case where the channel is not heated. For the heating channel, the factor 2 is missing!! ?? It is indeed pressure drop due to acceleration of the fluid, in the case where the channel is not heated. For the heating channel, the factor 2 is missing!!CONCLUSION: My opinion is that Bernoulli's law is applicable only for incompressible flows. Yet, when the fluid is heated, 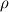 changes so that we cannot really speak about incompressibility. changes so that we cannot really speak about incompressibility.Do you see any mistake in my formulas or reasoning? Thank you for reading me. Jérôme |
|
|
|

|
|
 |
|
|
 Similar Threads
Similar Threads
|
||||
| Thread | Thread Starter | Forum | Replies | Last Post |
| Pressure Rise Error | emueller | CFX | 0 | May 5, 2009 11:08 |
| Pressure drop across a turbulent channel flow | shiv | Main CFD Forum | 0 | September 21, 2005 00:52 |
| Using ideal gas law to simulate pressure decline | Björn Mattsson | FLUENT | 5 | September 5, 2005 04:03 |
| what the result is negatif pressure at inlet | chong chee nan | FLUENT | 0 | December 29, 2001 05:13 |
| Definition of pressure | Ola Nordblom | FLUENT | 1 | August 16, 2001 15:58 |