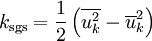Kinetic energy subgrid-scale model
From CFD-Wiki
The subgrid-scale kinetic energy is defined as
The subgrid-scale stress can then be written as
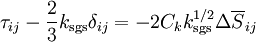
this gives us the transport equation for subgrid-scale kinetic energy
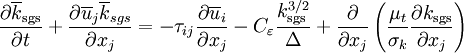
The subgrid-scale eddy viscosity,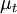 , is computed using
, is computed using  as
as