Near-wall treatment for LES models
From CFD-Wiki
The most basic form of wall modeling for LES simply imposes some additional constraints upon the eddy viscosity. The standard Smagorinsky model eddy viscosity is nonzero at solid boundaries, which is contrary to the notion that the eddy viscosity should be zero where there is no turbulence. The easy fix for this situation is to add a Van Driest-style damping function into the length scale:
Various different values for 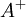 ,
, 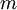 , and
, and 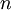 have been used. The use of this formulation requires the accurate computation of wall shear (in order to compute
have been used. The use of this formulation requires the accurate computation of wall shear (in order to compute 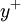 ), which has generally been accomplished by high grid resolution in near-wall regions. The legitimacy of terming such simulations as true LES's has been debated, as in the opinion of some the additional resolution is transitioning the simulation into a DNS in near-wall regions. At the very least, it is probably a violation of the "spirit" of LES, so models that do not require ad hoc damping have been sought.
), which has generally been accomplished by high grid resolution in near-wall regions. The legitimacy of terming such simulations as true LES's has been debated, as in the opinion of some the additional resolution is transitioning the simulation into a DNS in near-wall regions. At the very least, it is probably a violation of the "spirit" of LES, so models that do not require ad hoc damping have been sought.
The Zonal Two-layer Approach
The objective of the zonal strategy is to provide the LES region with the wall-shear stress, extracted from a separate modelling process applied to the near-wall layer. The wall-shear stress can be determined from an algebraic law-of-the-wall model or from differential equations solved on a near-wall-layer grid refined in
the wall-normal direction - an approach referred to as two-layer wall modelling. The method was originally proposed by Balaras and Benocci and tested by Tessicini et al.
At solid boundaries, the LES equations are solved up to a near-wall node which is located, typically, at 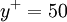 . From this node to the wall, a refined mesh is embedded into the main flow,and the following simplified turbulent boundary-layer equations are solved:
. From this node to the wall, a refined mesh is embedded into the main flow,and the following simplified turbulent boundary-layer equations are solved:
![\frac{\partial{\rho\tilde{U}_i}}{\partial{t}}+
\frac{\partial{\rho\tilde{U}_i\tilde{U}_j}}{\partial{x_j}}+
\frac{d\tilde{P}}{dx_i} =
\frac{\partial}{\partial{y}}[(\mu+\mu_t)\frac{\partial{\tilde{U}_i}}{\partial{y}}]\quad
i=1,3](/W/images/math/8/2/6/826c62fcb6d1a67d374b926431941843.png)
where y denotes the direction normal to the wall and i identify the wall-parallel directions (1 and 3).
The eddy viscosity 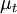 is
obtained from a mixing-length model with near-wall damping, as
done by Wang and Moin :
is
obtained from a mixing-length model with near-wall damping, as
done by Wang and Moin :
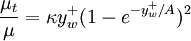 The boundary conditions for the turbulent boundary layer equations
are given by the unsteady outer-layer solution at the first grid node outside
the wall layer and the no-slip condition at y=0.
The boundary conditions for the turbulent boundary layer equations
are given by the unsteady outer-layer solution at the first grid node outside
the wall layer and the no-slip condition at y=0.
References:
Balaras E. and Benocci C. (1994) In: Applications of Direct and Large Eddy Simulation, AGARD. pp. 2-1-2-6.
Cabot W. and Moin P. (2000) Flow, Turbulence and Combustion, 63:269-291
Tessicini F., Temmerman L. and Leschziner M.A. (2005) In: 6th Engineering Turbulence Modelling and Measurements (ETMM6)
Add some text here about approximate boundary conditions, etc. Add references, too.

![D(y^+;A^+,m,n)=[1 - \exp(-{y^+}^n/{A^+}^n)]^m.](/W/images/math/0/9/6/0960020f19e325b771b7dfebca677a15.png)